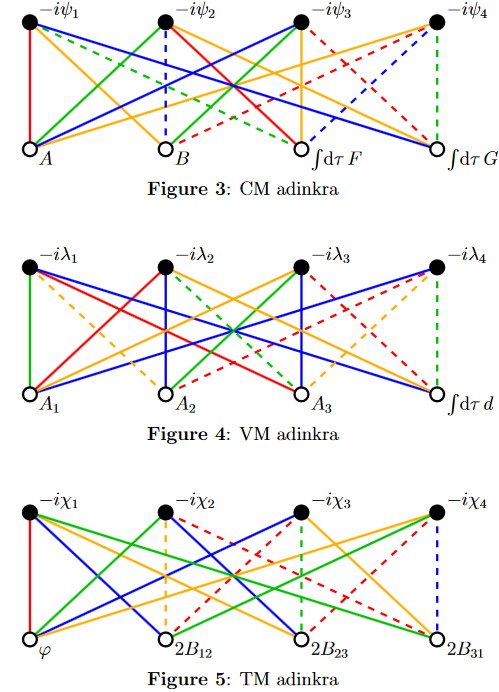
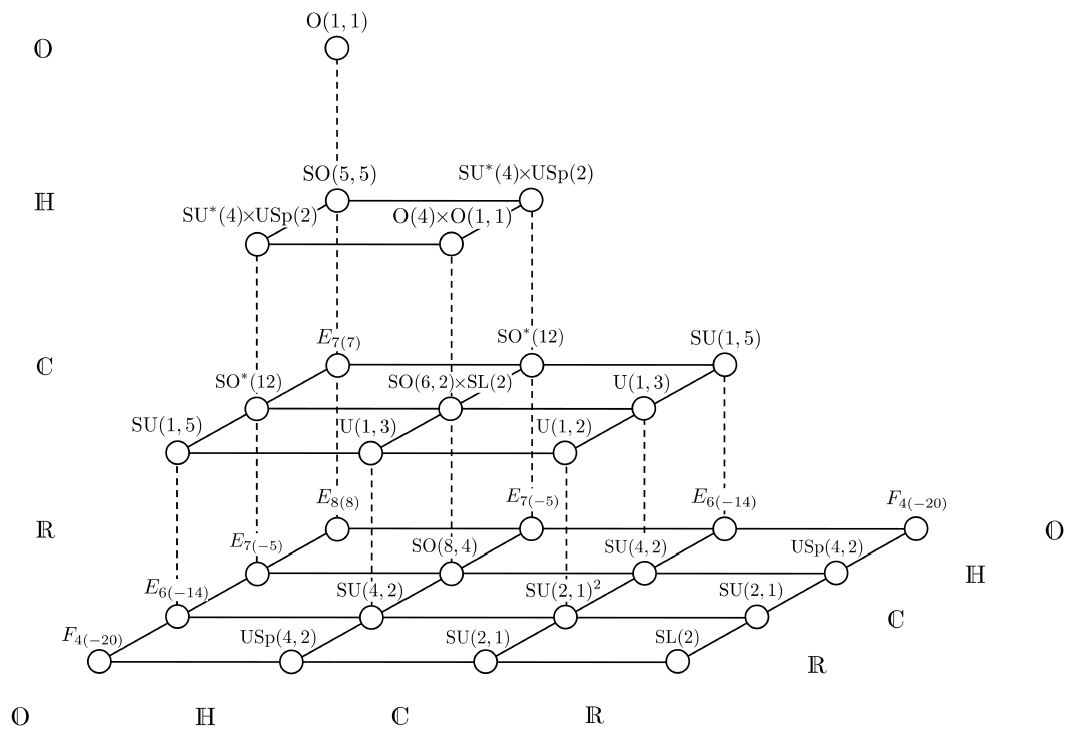
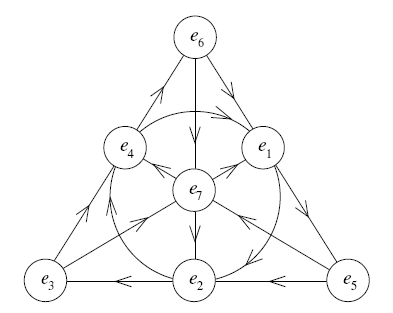
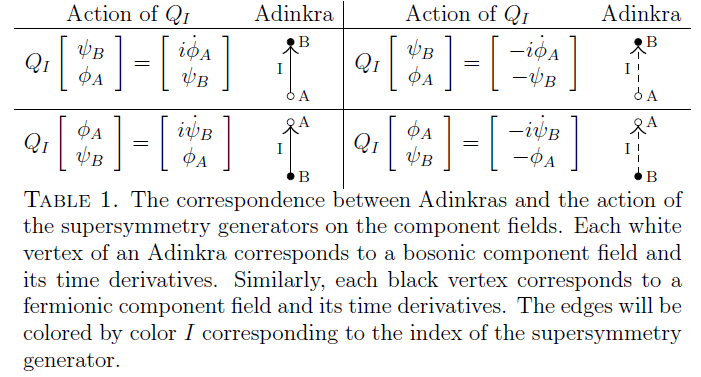
Division Algebra And Supersymmetry

26 Sep, 2021

LECTURES ON GEOMETRY OF SUPERSYMMETRY 5 2. The above noted associations of complex numbers and quaternions with supersymmetry can now be seen to form the following pattern.

Division Algebras And Supersymmetry Iii The N Category Cafe

SpinV of course is the component of PinV which lies in the even part of the Clifford algebra.

Division algebra and supersymmetry. There are exceptions however. Holds in spacetimes of dimensions three higherthan those of the division algebras. Supersymmetry is deeply related to division algebras.

Lie n-algebras supersymmetry and division algebras Introduction This research began as a puzzle. For example nonabelian YangMills fields minimally coupled to massless spinors are supersymmetric if and only if. The same is true for the Green-Schwarz superstring.

And supersymmetry in quantum field theory and string theory. The N 2 YM theory can also be written in a maximum of six dimensions. When W 567 mod 8 so the auxiliary spaces are symplectic we instead use N.

Let Cl V Q be the multiplicative group of units in ClV Q. Rex x x 2 Imx x x 2. Let x v1 v m then xˆ mv1 v.

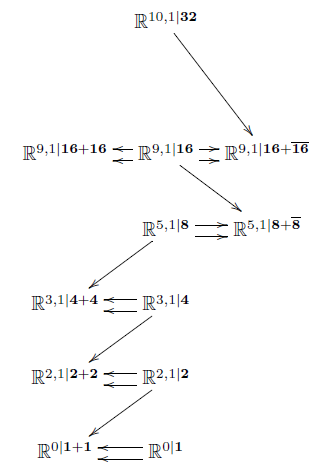
Starting from the four normed division algebras - the real numbers complex numbers quaternions and octonions - a systematic procedure gives a 3-cocycle on the Poincare Lie superalgebra in dimensions 3 4 6 and 10. Nonabelian Yang-Mills fields minimally coupled to massless spinors are supersymmetric if and only if the dimension of spacetime is 3 4 6 or 10. And this rule is why there exist supersymmetric 2-branes in these dimensions.

Its traditional to indicate a particular supersymmetry algebra using the dimension N or dimen-sions N N of the auxiliary spaces W or W W. Division Algebras and Supersymmetry According to Chapter 20 of Fulton and Harriss Representation Theory PinV is the set of x in CliffV such that x x 1 and x V x subseteq V. We begin with discussing the mathematical.

The real numbers R the complex numbers C the quaternions H and the octonions O. Supersymmetry in alternate numbers of dimensions. Supergravity theories seen as tensor products of super ang-MillsY theories are described as tensor products of division algebras leading to the identi cation of a magic square of supergrav-ity theories with their U-duality groups as the magic square entries.

I This allows us to deﬁne real and imaginary parts in the same way as for the complex numbers. They have dimensions k 1 2 4 and 8. Supersymmetry and Division Algebras Normed Division Algebras Let K be a normed division algebra of dimension n.

Nonabelian Yang-Mills fields minimally coupled to massless spinors are supersymmetric if and only if the dimension of spacetime is 3 4 6 or 10. Let x v1 v m then xt v v1. Division algebras are equivalent to objects called trialities each can be constructed from the other and the correspondence with Yang-Mills is achieved by showing that the condition for supersymmetry is equivalent to the gamma matrices decomposing to give a.

We deﬁne the following operations on Clifford algebras 1 Reﬂection automorphism. I The only normed division algebras are R C H and O. I The classical superstring makes sense only in dimensions k 2 3 4 6 and 10.

In d dimensions the size of spinors is approximately 2 d2 or 2 d 12. And truncation of supersymmetry are also described here by the four division algebras. 13 Notice that the transverse dimension D - 2 equals the dimension of the associated division algebra.

That is dimensions 4 5 7 and 11. Even better in the octonionic case that is 11-dimensional spacetime these 2-branes are closely related to 11-dimensional supergravity. The material is supplemented with material from Quantum Theory of Fields Vol III by Weinberg Modern Supersymmetry by John Ternin as well as a few of of my own additions.

A related procedure gives a 4-cocycle on the Poincare Lie superalgebra in dimensions 4 5 7 and 11. Because the properties of spinors change drastically between different dimensions each dimension has its characteristic. I The classical super-2-brane makes sense only in.

So you refer to things like the 3-dimensional N 2 supersymmetry algebra. Supersymmetry is deeply related to division algebras. The structure of the normed division algebras also governs the existence of the brane scan and the.

SPIN GROUP Deﬁnition 21. This is visible in the. Supersymmetry is deeply related to division algebras.

Then I K has a conjugation a linear operator satisfying x x xy yx. It is possible to have supersymmetry in dimensions other than four. Geometry of physics supersymmetry the section Real spin representations via Real alternative division algebra.

SUPERSYMMETRY LECTURE NOTES This is a set of lecture notes based primarily on a course given by Fernando Quevedo. Mathbb K one of the normed division algebras see at spin group The exceptional isomorphisms. We will introduce the classification of supersymmetry algebras using the division algebra perspective of Baez and Huerta BH10 which will be useful for the classification of super Yang-Mills.

The same is true for the Green-Schwarz superstring. There is a deep relation between supersymmetry and the four normed division algebras.

Normed Division Algebra In Nlab

Division Algebras And Supersymmetry Ii The N Category Cafe

Adinkra In Nlab

From The Superpoint To T Folds In Schreiber

Geometry Of Physics Supersymmetry In Nlab

Http Cbpfindex Cbpf Br Publication Pdfs Nf01301 2011 05 05 09 41 39 Pdf

Geometry Of Physics Fundamental Super P Branes In Nlab

Https Arxiv Org Pdf 1603 09063

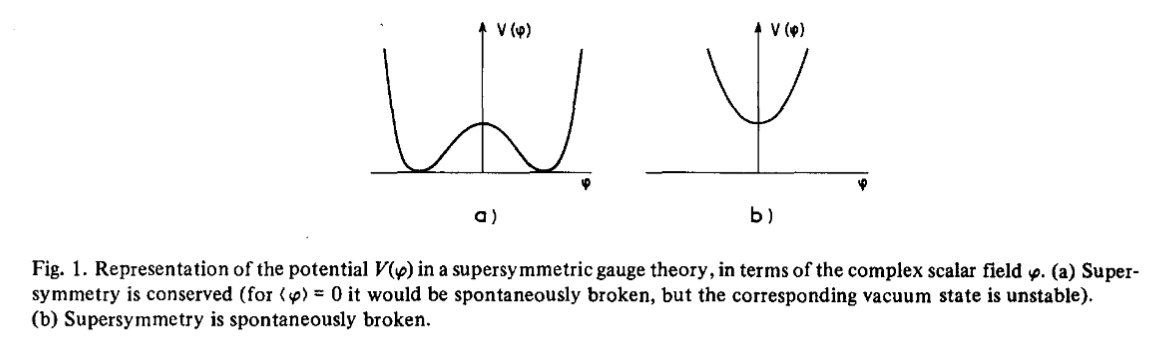
Supersymmetry Breaking In Nlab

Https Www Imperial Ac Uk Media Imperial College Research Centres And Groups Theoretical Physics Msc Dissertations 2013 Amel Durakovic Dissertation Pdf